Packing efficiency of metal crystal in simple cubic lattice :
Step 1 : Radius of sphere : In simple cubic unit cell, particles (spheres) are at the corners and touch each other along the edge.
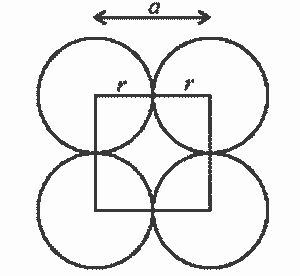
A face of simple cubic unit cell is shown in Fig. It is evident that
a = 2r or r = a/2 ………(1)
where r is the radius of atom and ‘a’ is the length of unit cell edge.
Step 2 : Volume of sphere : Volume of a sphere = .\(\frac{4π}{3}×r^3\)
Substitution for r from Eq. (1)
∴ Volume of one particle = \(\frac{4π}{3}(\frac{a}{2})^3\) = \(\frac{πa^3}{6}\)
Step 3 : Total volume of particles : Because simple cubic unit cell contains only one particle, volume occupied by particle in unit cell = \(\frac{πa^3}{6}\)
Step 4 : Packing efficiency
Packing efficiency = \(\frac{\text{Volume occupied by particles in unit cell}}{\text{total volume of unit cell}}×100\)
= \(\frac{πa^3/6}{a^3}×100 = \frac{π}{6}×100=\frac{3.142}{6}×100 \)
= 52.36%
Thus, in simple cubic lattice, 52.36 % of total space is occupied by particles and 100−52.36 = 47.64 % is empty space,
∴ void volume or a percentage of void space=.47.64 %